One Sample Z test is applied to test whether a sample mean in significantly different than a known / hypothesized mean, when sample size is more than 30 OR population standard deviation is known.
Steps:
1. Null hypothesis and Alternate Hypothesis
Null Hypothesis
A. Two tailed test: Sample mean is equal to hypothesized mean. OR Sample mean is not significantly different than hypothetical mean.
B. One tailed test: (Right tailed): Sample mean is not more than hypothesized mean. OR Sample mean is equal to or less than hypothesized mean.
C. One tailed test: (Left tailed): Sample mean is not less than hypothesized mean. OR Sample mean is equal to or more than hypothesized mean.
Alternate Hypothesis
A. Two tailed test: Sample mean is significantly different than hypothetical mean.
B. One tailed test: (Right tailed): Sample mean is significantly more than hypothesized mean.
C. One tailed test: (Left tailed): Sample mean is significantly less than hypothesized mean.
2. Calculate Test statistics Z
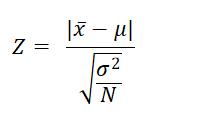
where Z is the test statistics,
x̄ = Sample mean,
μ = hypothesized / known / population mean,
σ =
Population Standard deviation. (If Sample Size is > 30, sample SD can be used as a measure of population SD)
N = Sample Size
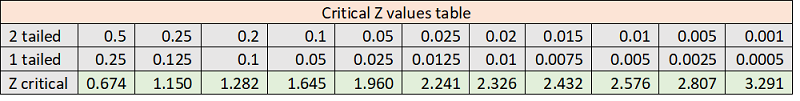
3. Know p value from Z table
4. Interpret
If p < = alpha, then reject Null hypothesis, and accept alternate hypothesis.
If p > alpha, then the study has failed to reject Null hypothesis. So accept Null hypothesis.
Confidence Intervals
1. For two tailed test:
100 - alpha % confidence interval = x̄ ± critical_Z_value * SE mean
where critical_Z_value is the Z table value corrsponding to 2 tailed test, given alpha and degrees of freedom.
SE mean = sqrt (σ2 / N)
1. For one tailed test:
A. Right tailed test (AH = Sample mean is significantly more than hypothesized mean)
100 - alpha % confidence interval = x̄ - critical_Z_value * SE mean to infinity.
where critical_Z_value is the Z table value corrsponding to 1 tailed test, given alpha and degrees of freedom.
B. Left tailed test (AH = Sample mean is significantly less than hypothesized mean)
100 - alpha % confidence interval = minus Infinity to x̄ + critical_Z_value * SE mean.
where critical_Z_value is the Z table value corrsponding to 1 tailed test, given alpha and degrees of freedom.
Example 1:
A sample of 50 premature birth children has shown mean IQ of 98 ± 5. Apply an approriate statistical test to test whether premature born children have significantly different IQ than population average of 100, at alpha level of 5%.
As N > 30, we can apply "One Sample Z test."
Here,
x̄ = 98
s = 5
μ = 100
N = 50
alpha = 5%
tails = 2 (significantly different)
Putting above values at the respective places, we get the output as follows:
p = 0.0047. P value is statistically significant at given alpha level of 0.05 (5%). Sample mean is significantly different than hypothesized / known mean.
Sample Mean ± SD = 98 ± 5
Hypothesized / known Mean = 100
Standard Error of Mean = 0.707
Z = 2.828
p = 0.0047(Two tailed)
Alpha = 0.05 (5 %). Critical Z value = 1.96
95 % Confidence interval = 96.614 to 99.386
Example 2:
A sample of 50 premature birth children has shown mean IQ of 98 ± 5. Apply an approriate statistical test to test whether premature born children have significantly less IQ than population average of 100, at alpha level of 5%.
As N > 30, we can apply "One Sample Z test."
Here,
x̄ = 98
s = 5
μ = 100
N = 50
alpha = 5%
tails = 1 (significantly less)
Putting above values at the respective places, we get the output as follows:
p = 0.0023. P value is statistically significant at given alpha level of 0.05 (5%). Sample mean is significantly less than hypothesized / known mean.
Sample Mean ± SD = 98 ± 5
Hypothesized / known Mean = 100
Standard Error of Mean = 0.707
Z = 2.828
p = 0.0023(One tailed)
Alpha = 0.05 (5 %). Critical Z value = 1.96
95 % Confidence interval = -∞ to 99.163
@ Sachin Mumbare