OR: Help

Aim: Statistical Power Calculation with given sample size: Hypothesis testing for Odds Ratio (RR) in case control study. Null Hypothesis: OR =1. Null hypothesis: RR =1

Formula Used for 2 tailed hypohesis

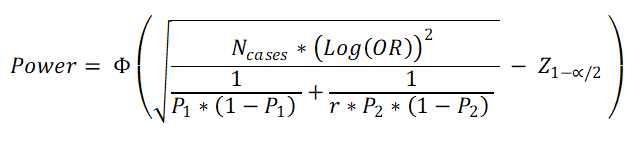
Formula Used for 1 tailed hypohesis

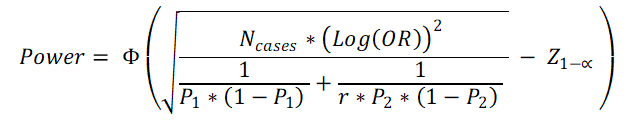
To calculate P1 or P2 or OR, when other two are known

P₁ = OR * P₂ / (1 - P₂ + OR * P₂)

P₂ = P₁ / (OR – OR * P₁ + P₁)

OR = P₁ * (1 – P₂) / (P₂ * (1 – P₁))

OR = Odds Ratio

P₁ = Proportion of Cases exposed to risk factor (out of 1) (e.g. 25% = 0.25)

P₂ = Proportion of Controls exposed to risk factor (out of 1) (e.g. 15% = 0.15)

N_cases = Sample Size in Case group

r = Controls to Cases ratio = N_controls / N_cases

α = 1 – Confidence level (out of 1) (e.g. 95% confidence level = 0.95, α = 0.05)

Z _1-α/2 = the standard normal deviate corresponding the confidence level

Φ(x)=P(Z ≤ x). It is the cumulative distribution function (CDF) of normal distribution. Simply, it is the area of the standard normal curve towards left side of x.

Example:

A case control study is coducted to test whether less attendance in class (exposure) is significantly associated with results in final examination (outcome). The study was conducted with 50 students in case (failure) group and 200 students in pass (controls) group. How much statistical power was achieved with this sampe size, if confidence level was 95 % (or alpha = 0.05)?

Solution:

Here

P₁ = 20%, P₂=5%, Confidence level = 95%, N_cases = 50, N_controls = 200, tails=2.

After putting these values, we get power = . 90.098 %.

We also get the estimated OR = 4.75.